This all-in-one online Capacitors in Series Calculator finds the capacitance of a circuit consisting of any number of capacitors connected in series. It can also find the capacitance of the capacitor that needs to be connected in series with the other capacitors to get the necessary total capacitance of this circuit.
You can enter the values of any known parameters in the input fields of this calculator and find the missing parameter. To add more capacitors you can click the “+” symbol on the right hand side. You can also click the “–” symbol to delete extra lines.
Capacitance in Series Formula
Capacitors are in series if they are connected in a row within an electrical circuit. Capacitors in series can be represented as the following diagram, where \(C_{1}\), \(C_{2}\), …, \(C_{n}\) are the capacitances of the capacitors connected in series.
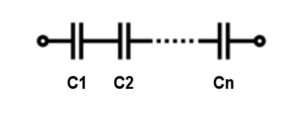
An important property of a circuit consisting of capacitors connected in series is that they have the same electric charge on their plates. This is easily explained by the fact that before the voltage source was connected to the circuit, all the capacitors had no charge. After connecting a voltage source, an electric charge appears on the capacitor plates. Moreover, it is obvious that the interconnected plates of adjacent capacitors will have the same magnitude, but different sign of charge.
Now, consider the voltages across the individual capacitors of our circuit. The voltage \(V_{i}\) across the capacitor \(i\) is expressed by the formula:
$$V_{i} = \frac{Q}{C_{i}},$$
where
\(Q\) is the electric charge in each capacitor,
\(C_{i}\) is the capacitance of the capacitor \(i\).
The total voltage \(V\) in the circuit is the sum of the individual voltages:
$$V = V_{1} + V_{2} + … + V_{n},$$
or using the above formula relating the voltage across a capacitor to its capacitance, we obtain the following expression:
$$\frac{Q}{C_{T}} = \frac{Q}{C_{1}} + \frac{Q}{C_{2}} + … + \frac{Q}{C_{n}},$$
where \(C_{T}\) is the total capacitance of the circuit.
Reducing the charge in this expression, we get the desired formula for the total capacitance of the circuit:
$$\frac{1}{C_{T}} = \frac{1}{C_{1}} + \frac{1}{C_{2}} + … + \frac{1}{C_{n}}.$$
In other words, the reciprocal of the total capacitance of all capacitors connected in series is equal to the sum of the reciprocals of the capacitances of these capacitors. It is easy to see that the total capacitance will always be less than the value of the smallest capacitance.
In practice, it is sometimes necessary to find the capacitance value of the capacitor that should be connected in series with the existing capacitor of known capacitance to get the necessary total capacitance.
The solution to any such problem can be easily found using our Capacitors in Series Calculator.
Related calculators
Check out our other physics calculators such as Ohm’s Law Calculator or Parallel Resistor Calculator.