This all-in-one online Inductors in Parallel Calculator finds the inductance of a circuit consisting of any number of inductors connected in parallel. It can also find the inductance of an inductor that needs to be connected in parallel with other inductors to get the necessary total inductance of this circuit.
You can enter the values of any known parameters in the input fields of this calculator and find the missing parameter. To add more inductors you can click the “+” symbol on the right hand side. You can also click the “–” symbol to delete extra lines.
Parallel Inductance Formula
Inductors are in parallel if they are connected to the same two points of an electrical circuit. Parallel inductors can be represented as the following diagram, where \(L_{1}\), \(L_{2}\), …, \(L_{n}\) are the inductance values of the inductors connected in parallel.
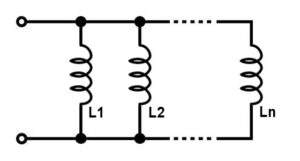
An important property of a circuit consisting of inductors connected in parallel is that they share the same voltage \(V\) on their terminals. Further, according to Kirchhoff’s current law, the total electric current through the circuit \(I_{T}\) is equal to the sum of the individual currents flowing through each inductor \(I_{i}\):
$$I_{T} = I_{1} + I_{2} + … + I_{n}.$$
On the other hand, we know that the self-induced voltage across an inductor is given by the expression:
$$V = L_{i} \frac{dI_i}{dt},$$
where \(\frac{dI_i}{dt}\) is the rate of change of current through the inductor \(i\).
Further, differentiating with respect to time the above expression for the total current in the circuit and using the relation
$$\frac{V}{L_{i}} = \frac{dI_i}{dt},$$
we obtain the following expression:
$$\frac{V}{L_{T}} = \frac{V}{L_{1}} + \frac{V}{L_{2}} + … + \frac{V}{L_{n}}.$$
Dividing both parts of this expression by the value of the common voltage \(V\), we obtain the following result:
$$\frac{1}{L_{T}} = \frac{1}{L_{1}} + \frac{1}{L_{2}} + … + \frac{1}{L_{n}}.$$
In other words, the reciprocal of the total inductance of all inductors connected in parallel is equal to the sum of the reciprocals of the inductances of these inductors. It is easy to see that the total inductance will always be less than the value of the smallest inductance.
In practice, it is sometimes necessary to find the inductance value of an inductor that should be connected in parallel with the existing inductor of known inductance to get the necessary total inductance.
The solution to any such problem can be easily found using our Inductors in Parallel Calculator.
Related calculators
Check out our other physics calculators such as Inductive Reactance Calculator or Parallel Capacitor Calculator.